Strain and transport properties in AlGaN nanocolumns with a GaN Quantum Disk
In the last couple of years a huge effort has been devoted to achieve and to control the growth of III-nitride columnar-shaped nanostructures: nanorods, nanocolumns, nanopillars. Results have shown, so far, an extremely high crystal quality of the AlGaN nanocolumns, that are strain-free and have no dislocations or other extended defects, thus, yielding an outstanding emission efficiency. The achievement of nanocolumnar heterostructures including quantum disks (QDisks) and nanocavities with QDisks and Bragg mirrors have been reported.
With TiberCAD package, a full 3D model of an AlGaN nanocolumn heterostructure with a 4.5 nm-wide GaN quantum disk has been designed and used to perform calculations of strain and transport properties.

The geometrical model of the nanocolumn has been constructed and meshed both with the ISE-TCAD tool DEVISE and with the open-source GMSH software.
Then, calculations have been performed with TiberCAD by applying the following physical models:
1) linear Strain model for the calculation of strain tensor, displacements, deformation potentials and piezoelectric charge at the AlGaN/GaN interfaces.
2) Poisson-Drift-Diffusion model for calculation of transport of electron and holes in ananocolumn-based p-i-n diode.
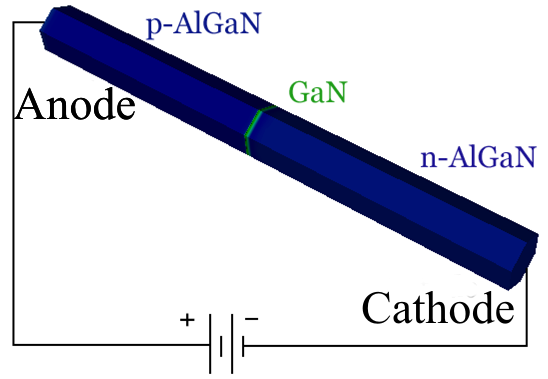
The nanocolumn has been contacted with a anode (p-contact) and a cathode (n-contact) and a pin diode has been modelled by doping the two AlGaN regions with a 1019 cm-3 concentration of acceptors (Mg, with 0.17eV energy level) and donors (Si) respectively.
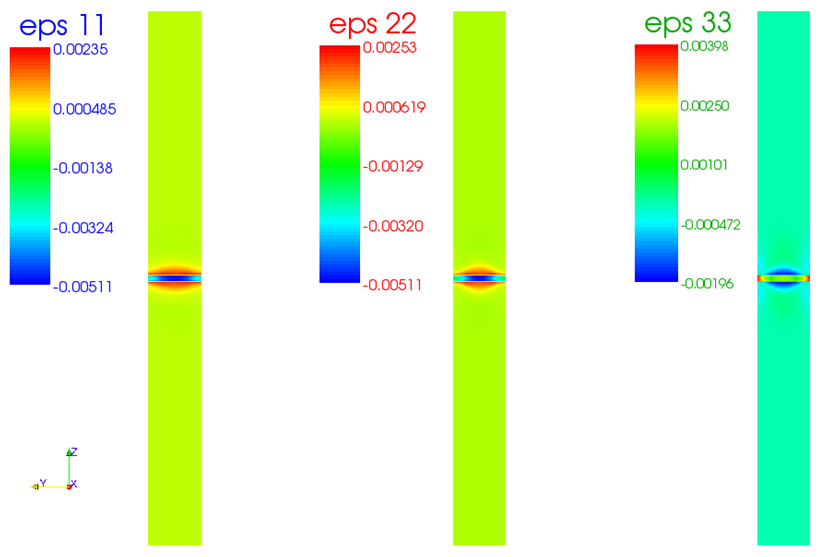
The strain tensor components show that GaN quantum disk is subjected to compressive strain in the in-plane directions, since GaN lattice constant is larger than the one of AlGaN, which is taken in this case as a relaxed reference layer. On the contrary, strain is tensile in orthogonal direction.
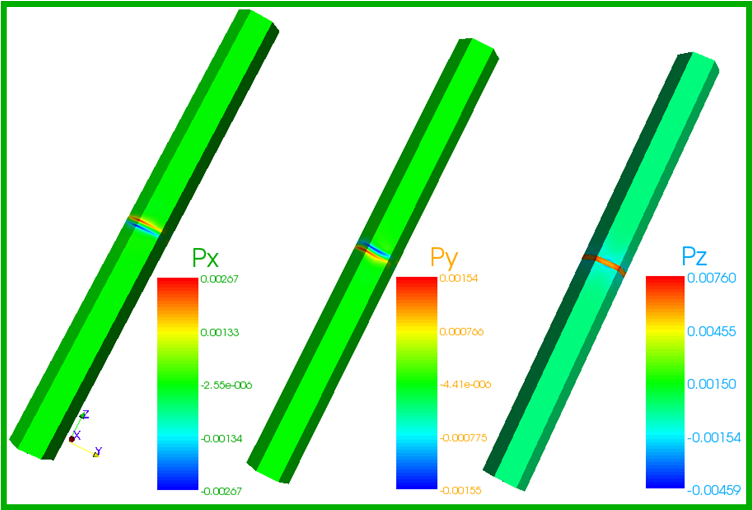
Piezoelectric polarization, mostly localized at AlGaN/GaN interfaces, is shown also; the sum of piezoelectric and spontaneous (pyro) polarization is included in the subsequent Poisson-Drift-Diffusion calculation.
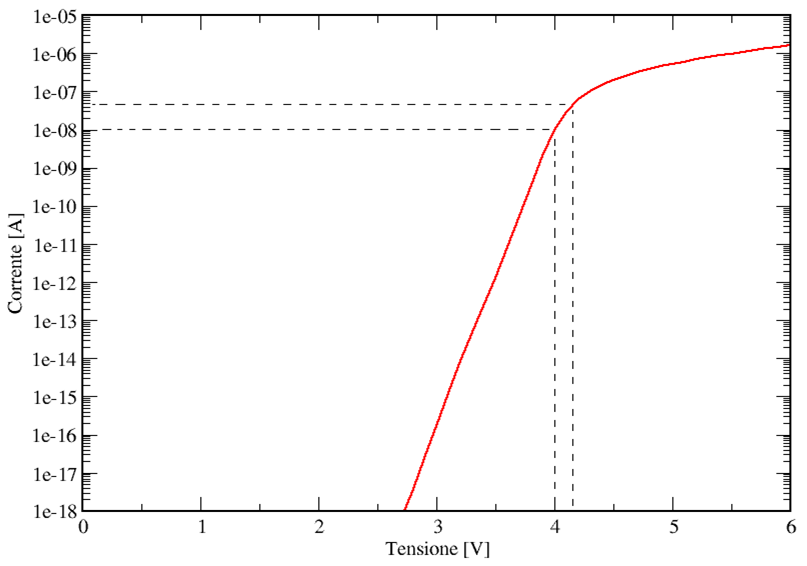
The I-V characteristic for the diode is finally obtained by solving Poisson and Drift-Diffusion using a Newton type algorithm.
References:
- G.Parrella, "Simulazione di dispositivi basati sul nitruro di gallio", thesis, 2007
- L. Cerutti, J. Ristić, S. Fernández-Garrido, E. Calleja , A. Trampert, K. H. Ploog, S. Lazic and J. M. Calleja, "Wurtzite GaN nanocolumns grown on Si (001) by molecular beam epitaxy", Appl. Phys. Lett. 88, 213114, (2006)
- J. Ristia, E. Calleja, M. A. Sanchez-Garcıa, J. M. Ulloa, J. Sanchez-Paramo , J. M. Calleja, U.Jahn, A. Trampert, and K. H. Ploog, "Characterization of GaN quantum discs embedded in AlxGa(1-x)N nanocolumns grown by molecular beam epitaxy" , Phys. Rev. B 68, 125305, (2003)


